The article/blog is being written by George Schuetz. It is being reproduced here.
But how are you going about it? Some machinists, with experience using gage blocks, will begin creating the desired dimension by starting with the largest block first and grabbing blocks as they go. Then they get stuck and start wringing their hands.
However, there’s more to combining gage blocks than just “stacking” blocks. When done properly, you can create any dimension from zero to four inches, to the nearest tenth of a thousandth, never using more than four blocks or never using more than one from each series of blocks. In addition, you are assured of minimizing wringing error and randomly spreading the usage among all the blocks, thus reducing wear on the blocks.
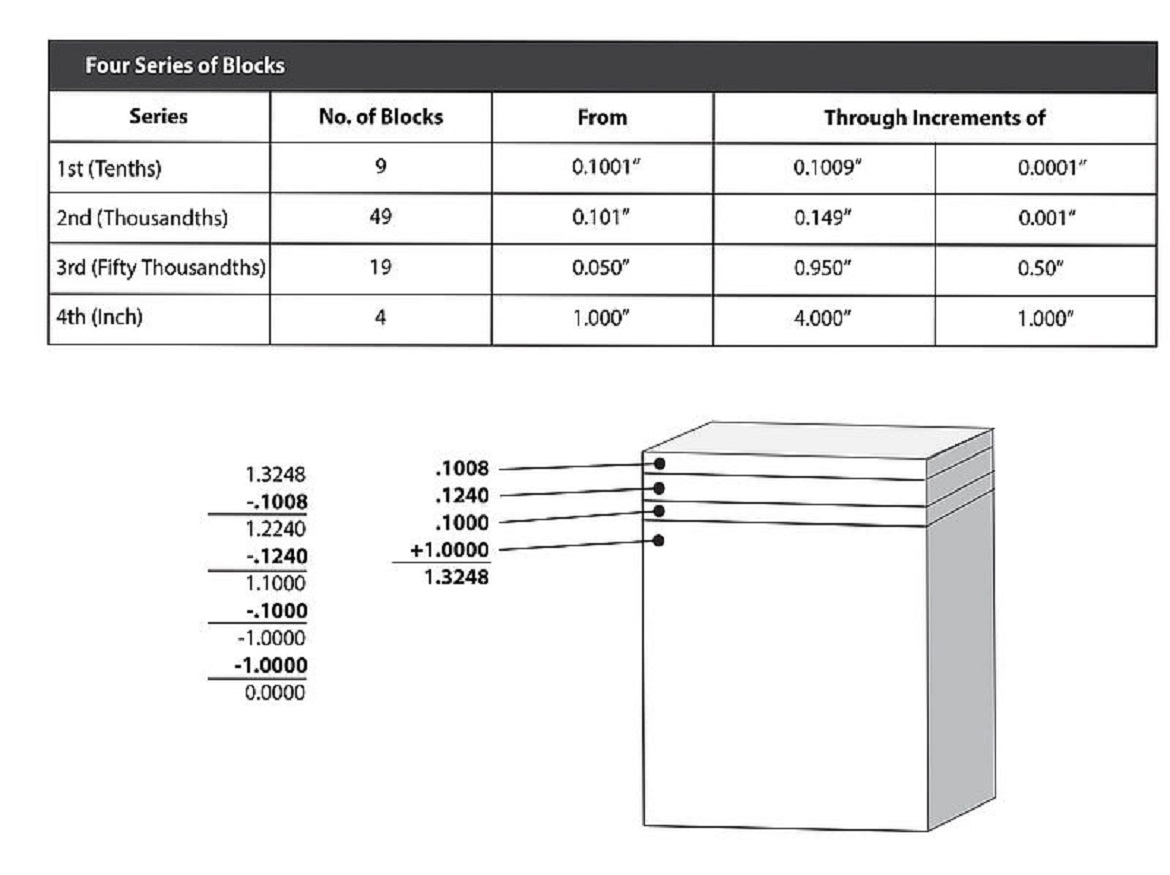
Let’s say the dimension we need is 1.3248 inches. We’ve got four series of blocks, as shown in Table 1. Now, to create 1.3248 inches, rather than saying, “I need a one inch block,” we start at the right side of the dimension and work backwards, with the goal of creating zeros all the way across.
To get rid of the 8, there’s only one series with an 8 in the fourth place: the Tenths. So we select the 0.1008-inch block. Subtract from 1.3248 inches, we’re left with 1.2240 inches.
Now we want to get rid of a 4 in the third place. We have more than one in the Thousandths Series blocks with a 4 in the third place-0.104 inch, 0.114 inch, 0.124 inch, 0.134 inch and 0.144 inch. To get rid of the 4, as well as the 2 in the second place, we select the 0.124-inch block. Subtracted from 1.2240 inches and we are left with 1.1000 inches. See how quickly this system gives us round numbers.
We have a 0.1000 block in the Fifty Thousandths Series, which leaves us with 1.0000 when subtracted from our number. Finally, to get rid of the one-inch number, we have the one-inch block in the Inch Series. So, the math for 1.3248 inches looks like that shown in the example above.
By working from right to left, we have created zeros all the way across. We have not used more than four blocks, and only used one block from each series.
We could create the same dimension using many more blocks, but there are only three “wrings” in a stack of four blocks. There could easily be wringing error of four-millionths per wring. The more blocks we use, the greater the wringing error, and the more the blocks are exposed to the shop environment, where they may get dirty, scratched or lost. We need to combine gage blocks properly for the same reasons we need to give them lots of care.